Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.
Ideal Gas Equation
The gaseous state is described completely using the following four variables T, P, V and n and their relationships were governed by the gas laws studied so far.
Boyle’s law V α \(\frac{1}{P}\)
Charles law V α T
Avogadro’s law V α n
We can combine these equations into the following general equation that describes the physical behaviour of all gases.
V α \(\frac{nT}{P}\)
where, R is the proportionality constant called universal gas constant.
The above equation can be rearranged to give the ideal gas equation
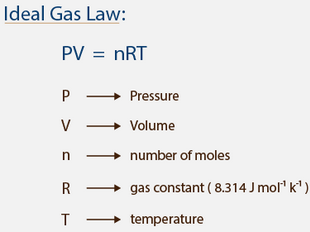
PV = nRT …………………. (6.11)
We already know that pressure is expressed in many different units (Table 6.1) hence it is important to know the values of gas constant R in different units as well.
We can calculate R using the equation,
R = \(\frac{PV}{nT}\)
For Conditions in which P is 1 atm., volume 22.414 dm3 for 1 mole at 273.15 K.

Under standard conditions (STP) Where P = 1 bar (105 pascal), V= 22.71 × 10-3m3 for 1 mole
of a gas at 273.15 K
![]()
= 8.314 Pa m3K-1mol-1
= 8.314 × 10-5bar m3K-1mol-1
= 8.314 × 10-2bar dm3K-1mol-1
= 8.314 × 10-2bar L K-1mol-1
= 8.314 J K-1mol-1
The ideal gas equation is a relationship between four variables (P, V, T, n). Since it describes the state of any gas, it is referred to as the equation of state of gases.
Let us calculate the pressure exerted by 2 moles of sulphur hexafluoride in a steel vessel of volume 6 dm3 at 70 °C assuming it is an ideal gas. We will use the ideal gas equation for this calculation as below:

= 9.39 atm.
The ideal gas law states that PV = NkT, where P is the absolute pressure of a gas, V is the volume it occupies, N is the number of atoms and molecules in the gas, and T is its absolute temperature.
In chemistry, the formula PV=nRT is the state equation for a hypothetical ideal gas. In the equation PV=nRT, the term “R” stands for the universal gas constant.
In SI units, p is measured in pascals, V is measured in cubic metres, n is measured in moles, and T in kelvins (the Kelvin scale is a shifted Celsius scale, where 0.00 K = -273.15 °C, the lowest possible temperature). R has the value 8.314 J/(K⋅mol) ≈ 2 cal/(K⋅mol), or 0.0821 L⋅atm/(mol⋅K).
It is the combination of Boyle’s law, Charles’s law and Avogadro’s law PV/T = constant the value of constant depends on for amount of gas and the units in which pressure and volume are measured. … (c) PV = nRT. PV =m/M × RT. The equation is called as an ideal gas equation.
Ideal Gas and Non-Ideal Gas Equation
Two types of gases exist. Real gas and Ideal gas. As the particle size of an ideal gas is extremely small and the mass is almost zero and no volume Ideal gas is also considered as a point mass. The molecules of real gas occupy space though they are small particles and also have volume.
The gas particles have negligible volume. The gas particles are equally sized and do not have intermolecular forces (attraction or repulsion) with other gas particles. The gas particles move randomly in agreement with Newton’s Laws of Motion. The gas particles have perfect elastic collisions with no energy loss. Ideal gases have mass and velocity.
Real Gas:
Real gases are defined as the gases that do not obey gas laws at all standard pressure and temperature.
The gas laws consist of three primary laws:
Charles’ Law, Boyle’s Law and Avogadro’s Law (all of which will later combine into the General Gas Equation and Ideal Gas Law).
An ideal gas is a gas whose pressure P, volume V, and temperature T are related by the ideal gas law: PV = nRT. where n is the number of moles of the gas and R is the ideal gas constant. Ideal gases are defined as having molecules of negligible size with an average molar kinetic energy dependent only on temperature.
Since the particles of an ideal gas have no volume, a gas should be able to be condensed to a volume of zero.
Reality Check:
Real gas particles occupy space. A gas will be condensed to form a liquid which has volume. The gas law no longer applies because the substance is no longer a gas.