CBSE Sample Papers for Class 10 Maths Standard Set 1 with Solutions

Students can access the CBSE Sample Papers for Class 10 Maths Standard with Solutions and marking scheme Set 1 will help students in understanding the difficulty level of the exam.

CBSE Sample Papers for Class 10 Maths Standard Set 1 with Solutions

Time Allowed: 3 hours
Maximum Marks: 80

General Instructions:

1. This Question Paper has 5 Sections A, B, C, D and E.
2. Section A has 20 MCQs carrying 1 mark each
3. Section B has 5 questions carrying 02 marks each.
4. Section C has 6 questions carrying 03 marks each.
5. Section D has 4 questions carrying 05 marks each.
6. Section E has 3 case based integrated units of assessment (04 marks each) with sub parts of the values of 1, 1 and 2 marks each respectively.
7. All Questions are compulsory. However, an internal choice in 2. Qs of 5 marks, 2.Qs of 3 marks and 2. Questions of 2. marks has been provided. An internal choice has been provided in the 2 marks questions of Section E.
8. Draw neat figures wherever required. Take n =22/7 wherever required if not stated.

Section – A ( 20 Marks) 
Section A Consists of Multiple Choice Type questions of 1 mark each

Question 1.

If two positive integers a and b are written as a = x³y² and b = xy³, where x, y are prime numbers, then the result obtained by dividing the product of the positive integers by the LCM (a, b) is
(A) xy
(B) xy²
(C) x³y³
(D) x²y²
Solution:
(B) xy²

Detailed Answer:
Given, a = x³y² and b = xy³
LCM (a, b) = x³y²
Product (A) and (B) = x³y² x xy³
Dividing product (a, b) by LCM (a, b)
\( \frac{\text { Product } a \text { and } b}{\text { LCM }} \) = \( \frac{x^3 y^2 \times x y^3}{x^3 y^3} \) = xy²

Question 2.
The given linear polynomial y = f(x) has [1]

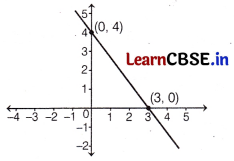
(a) 2 zeroes
(b) 1 zero and the zero is ‘3 ’
(c) 1 zero and the zero is ‘4’
(d) No zero
Answer:
(b) 1 zero and the zero is ‘3’

Since, the given polynomial y = f(x) intersects the X-axis at point (3, 0) only. So, the polynomial has 1 zero and the zero is 3.

Question 3.
The lines representing the given pair of linear equations are non-intersecting. Which of the following statements is true?

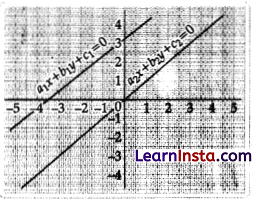
(A) \(\frac{a_1}{a_2}=\frac{b_1}{b_2}=\frac{c_1}{c_2}\)
(B) \(\frac{a_1}{a_2}=\frac{b_1}{b_2} \neq \frac{c_1}{c_2}\)
(C) \(\frac{a_1}{a_2} \neq \frac{b_1}{b_2}=\frac{c_1}{c_2}\)
(D) \(\frac{a_1}{a_2} \neq \frac{b_1}{b_2} \neq \frac{c_1}{c_2}\)
Solution:
(B) \(\frac{a_1}{a_2}=\frac{b_1}{b_2} \neq \frac{c_1}{c_2}\)

Detailed Answer:
Two pair of linear equations intersect so there line is parallel lines, there are no solution
\(\frac{a_1}{a_2}=\frac{b_1}{b_2} \neq \frac{c_1}{c_2}\)

Question 4.
Write the nature of roots of the quadratic equation 9x² – 6x – 2 = 0 [1]
(a) no real roots
(b) 2 equal real roots
(c) 2 distinct real roots
(d) more than 2 real roots
Answer:
(c) 2 distinct real roots

Given, 9x² – 6x – 2 = 0
Discriminant, D = (-6)² – 4(9) (- 2)
= 36 + 72 = 108 > 0
∴ The given equation has 2 distinct real roots.

Question 5.
Two APs have the same common difference. The first term of one of these is – 1 and that of the other is – 8. The between their 4th terms is:
(A) 1
(B) -7
(C) 7
(D) 8
Solution:
(C) 7

Detailed Answer:
Given two APs and their first term are -1 and -8 and have same common difference.
Since, n^th term is given as T_n = a + (n – 1)d
Now, 4^th term of first AP
T₄ = (-1) + (4 – 1)d
T₄ = -1 + 3d …(i)
4th term of second AP
T₄^’ = -8 + 3d …(ii)
Subtract eq (ii) from eq (i)
T₄ – T₄^’ = (-1 + 3d) – (-8 + 3d)
T₄ – T₄^’ = 7
Hence the difference between their 4th term is 7.

Question 6.
The ratio in which the line segment joining (2, -3) and (5, 6) is divided by X-axis [1]
(a) 1 : 2
(b) 2 : 1
(c) 2 : 5
(d) 5 : 2
Answer:
(a) 1 : 2

Let the point on X-axis which divides the join of (2, – 3) and (5, 6) be (x, 0).
Let the line segment be divided by (x, 0) in the ratio k : 1.
Using section formula,
0 = \(\frac{k(6)+1(-3)}{k+1}\)
⇒ 6k = 3
⇒ k = \(\frac{1}{2}\)

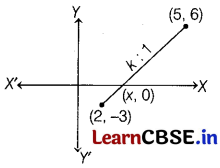
∴ The ratio is k : 1 = \(\frac{1}{2}\) : 1 = 1 : 2

Question 7.
A point (x, y) is at a distance of 5 units from the origin. pane such points lie in the third quadrant?
(A) 0
(B) 1
(C) 2
(D) infinitely many
Solution:
(D) infinitely many

Detailed Answer:
(x, y) is 5 units from the origin. There are infinity many point in from origin between point (x, y).

Question 8.
In ∆ABC, DE || AB. If AB = a, DE = x, BE = b, and EC = c. Express x in terms of a, b, and c [1]

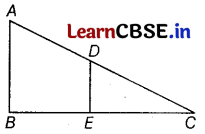
(a) \(\frac{a c}{b}\)
(b) \(\frac{a c}{b+c}\)
(c) \(\frac{a b}{c}\)
(d) \(\frac{a b}{b+c}\)
Answer:
(b) \(\frac{a c}{b+c}\)

In ∆CED and ∆CBA,
∠CED = ∠CBA [corresponding angles, ∵ DE || AB and CB is the transversal]
∠C is the common angle.
∴ ∆CED ~ ∆CBA [by AA similarity criterion]
⇒ \(\frac{C E}{C B}=\frac{E D}{B A}\)
⇒ \(\frac{c}{c+b}=\frac{x}{a}\)
⇒ x = \(\frac{a c}{b+c}\)

Question 9.

If O is centre of a circle and chord PQ makes an angle 50° with the one a tangent PR at the point of contact P, then the angle subtended by the chord at the centre is:
(A) 130°
(B) 100°
(C) 50°
(D) 30°
Solution:
(B) 100°

Detailed Answer:

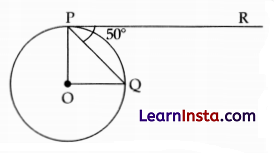
Given ∠QPR = 50°
PQ is a tangent, so ∠OPR is 90°.
Then, ∠OPR = ∠OPQ + ∠QPR
90° = ∠OPQ + 50°
40° = ∠OPQ = ∠OQP  (radii of the circle)
In ΔOQP
∠POQ + ∠OQP + ∠OPQ = 180°
∠POQ + 40° + 40° = 180°
∠POQ = 100°

Question 10.
A quadrilateral PQRS is drawn to circumscribe a circle. If PQ =12 cm, QR = 15 cm and RS = 14 cm, then the length of SP is [1]
(a) 15 cm
(b) 14 cm
(c) 12 cm
(d) 11 cm
Answer:
(d) 11 cm

A quadrilateral PQRS is drawn to circumscribe a circle. As shown in figure.

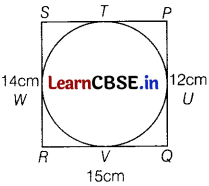
Let T, U, V and W be the points of contact of the line segment SP, PQ, QP and RS, respectively.
Now, PT = PU ………..(i)
[tangents from an external point]
ST = WS ………….(ii)
RW = RV ⇒ PV = RW ………(iii)
QU = QV ⇒ QV = QU ………(iv)
Adding Eqs. (i), (ii), (iii) and (iv), we get
PT+ ST + RV+ OV = PU + WS + RW + QU
⇒ (PT + ST) + (RV + QV) = (PU + QU) + (WS + WR)
⇒ SP + RQ = PQ + RS
⇒ SP + 15 = 12 + 14
⇒ SP = 11 cm

Question 11.
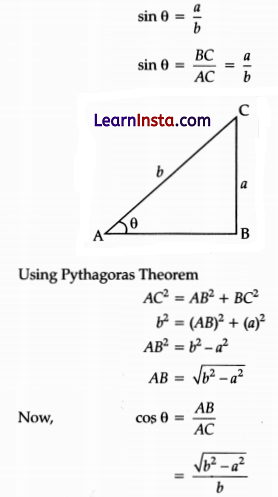
Given that sin θ = \(\frac{a}{b}\), find cos θ is:
(A) \(\frac{b}{\sqrt{b^2-a^2}}\)
(B) \(\frac{b}{a}\)
(C) \(\frac{\sqrt{b^2-a^2}}{b}\)
(D) \(\frac{a}{\sqrt{b^2-a^2}}\)
Solution:

(C) \(\frac{\sqrt{b^2-a^2}}{b}\)

Detailed Answer:

Question 12.
(sec A + tan A) (1 – sin A) is equal to [1]
(a) sec A
(b) sin A
(c) cosec A
(d) cos A
Answer:
(d) cos A

We have, (sec A + tan A) (1 – sin A)
= sec A – sec A sin A + tan A – tan A sin A
= sec A – tan A + tan A – tan A sin A
= \(\frac{1}{\cos A}-\frac{\sin ^2 A}{\cos A}\) = \(\frac{1-\sin ^2 A}{\cos A}\)
= \(\frac{\cos ^2 A}{\cos A}\) = cos A

Question 13.
If a pole 6 m high casts a shadow 23 m long on the ground, then the Sun’s elevation is:
(A) 6°
(B) 45°
(C) 30°
(D) 90°
Solution:
(A) 6°

Detailed Answer:
Let the pole AC is 6 m and shadow AB is 2√3 m.

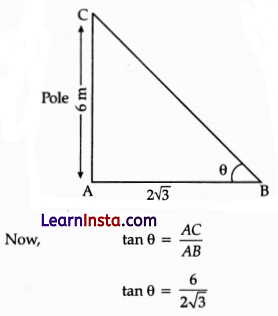
tan θ = √3
tan θ = tan 60°
θ = 60°

Question 14.
If the perimeter and the area of a circle are numerically equal, then the radius of the circle is [1]
(a) 2 units
(b) n units
(c) 4 units
(d) 7 units
Answer:
(a) 2 units

Let the radius of a circle be r.
Given, 2πr = πr²
⇒ r² – 2r = 0
⇒ r(r – 2) = 0
r = 0 or r = 2
Since the radius can not be 0. Therefore, r = 2 units.

Question 15.
It is proposed to build a new circular park equal in area to the sum of areas of two circular parks of diameters 16 m and 12 m in a locality. The radius of the new park is:
(A) 10 m
(B) 15 m
(C) 20 m
(D) 24 m
Solution:
(A) 10 m

Detailed Answer:
Let radius of new park be R.
r₁ = 8 cm
r₂ = 6 m
Now, Area of new park = Sum of area of two circular parks πR² = πr₁² + πr₂²
πR² = π(r₁² + r₂²)
R² = 64 + 36
R = 10 m

Question 16.
There is a green square board of side ‘2 a’ unit circumscribing a red circle. Jayadev is asked to keep a dot on the above said board. Then, the probability that he keeps the dot on the green region is [1]
(a) \(\frac{\pi}{4}\)
(b) \(\frac{4-\pi}{4}\)
(c) \(\frac{\pi-4}{4}\)
(d) \(\frac{4}{\pi}\)
Answer:
(b) \(\frac{4-\pi}{4}\)

Given, the side of a green square board is 2a.

Radius of the circle = \(\frac{2 a}{2}\) = a units
∴ Area of the square board = (2a)² = 4a² sq units
∴ Area of red region = Area of circle = ita2 sq units
∴ Area of green region = Area of the square board – Area of red region
= (4a² – πa²)sq units
∴ Probability (keeping the dot on the green region)
= \(\frac{\text { Area of the green region }}{\text { Area of the square board }}\)
= \(\frac{4 a^2-\pi a^2}{4 a^2}\) = \(\frac{4-\pi}{4}\)

Question 17.
2 cards of hearts and 4 cards of spades are missing from a pack of 52 cards. A card is drawn at random from the remaining pack. What is the probability of getting a black card?
(A) \(\frac{22}{52}\)
(B) \(\frac{22}{46}\)
(C) \(\frac{24}{52}\)
(D) \(\frac{24}{46}\)
Solution:
(B) \(\frac{22}{46}\)

Detailed Answer:
Given, 2 cards of hearts and 4 cards are missing then,
Remaining total cards = 52 – 6
n(S) = 46
Total black cards = 26
remaining black cards n(E) = 26 – 4
= 22
Probability (getting black cards)
= \(\frac{n(E)}{n(S)}\)
= \(\frac{22}{46}\)

Question 18.
The upper limit of the modal class from the given distribution. [1]

Height [in cm]	Below 140	Below 145	Below 150	Below 155	Below 160	Below 165
Number of girls	4	11	29	40	46	51

(a) 165
(b) 160
(c) 155
(d) 150
Answer:
(d) 150

Let us make the table for the given distribution

Height (in cm)	Class-interval (in cm)	Cumulative frequency	Frequency
Below 140	0-140	4	4
Below 145	140-145	11	7
Below 150	145-150	29	18
Below 155	150-155	40	11
Below 160	155-160	46	6
Below 165	160-165	51	5

∴ The modal class is 145-150 and the upper limit of the model class is 150.

DIRECTION: In questions number 19 and 20, a statement of assertion (A) is followed by a statement of Reason (R).
Choose the correct option.

Question 19.
Statement A (Assertion): Total Surface area of the top is the sum of the curved surface area of the hemisphere and the curved surface area of the cone.
Statement R (Reason): Top is obtained by joining the plane surfaces of the hemisphere and cone together.
(A) Both assertion (A) and reason (R) are true and reason (R) is the correct explanation of assertion (A)
(B) Both Assertion (A) and reason (R) are true and reason (R) is not the correct explanation of assertion (A)
(C) Assertion (A) is true but reason (R) is false.
(D) Assertion (A) is false but reason (R) is true.
Solution:
(A) Both assertion (A) and reason (R) are true and reason (R) is the correct explanation of assertion (A)

Detailed Answer:
Total surface area of top = Curved surface of cone + Curved surface area of hemisphere
Assertion is true.
Reason: Top is obtained by fixing the plane surface of hemisphere and cone together The flat surface of the cone and hemisphere will be contact with each other when they are fixed together to form the top.
Reason is true.
Both assertion and reason are true and reason (R) is correct explanation of assertion (A).

Question 20.
Assertion (A) : -5, \(\frac{-5}{2}\), 0, \(\frac{5}{2}\), …….. is in Arithmetic Progression.
Reason (R) : An Arithmetic Progression cannot have both positive and negative rational numbers. [1]
(a) Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).
(b) Both Assertion (A) and Reason (R) are true and Reason (R) is not the correct explanation of Assertion (A).
(c) Assertion (A) is true but Reason (R) is false.
(d) Assertion (A) is false but Reason (R) is true.
Answer:
(c) Assertion (A) is true but Reason (R) is false.

Given progression is -5, \(\frac{-5}{2}\), 0, \(\frac{5}{2}\), ……..
Here, first term a₁ = -5,
second term a₂ = \(\frac{-5}{2}\),
third term a₃ = 0,
and fourth term a₄ = \(\frac{5}{2}\)
Now,
a₂ – a₁ = \(\frac{-5}{2}\) – (-5)
= \(\frac{-5}{2}\) + 5 = \(\frac{5}{2}\)
a₃ – a₂ = 0 – \(\frac{-5}{2}\) = \(\frac{5}{2}\)
a₄ – a₃ = \(\frac{-5}{2}\) – 0 = \(\frac{5}{2}\)
∵ Common difference between each term is \(\frac{5}{2}\)
∴ Assertion (A) is true.

Section – B (10 Marks)
Section B consists of 5 questions of 2 marks each.

Question 21.
Prove that √2 is an irrational number.
Solution:
Let us assume, to the contrary, that √2 is rational.
So, we can find integers a and b such that √2 = a/b
where a and b are coprime.
So, b√2 = a
Squaring both sides, we get 2b² = a²
Therefore, 2 divides a² and so 2 divides a.
So, we can write a = 2c for some integer c.
Substituting for a, we get 2b² = 4c², that is, b² = 2c².
This means that 2 divides b², and so 2 divides b.
Therefore, a and b have at least 2. as a common factor.
But this contradicts the fact that a and b have no common factors other than 1.
This contradiction has arisen because of our incorrect assumption that √2 is rational.
So, we conclude that √2 is irrational.

Question 22.
ABCD is a parallelogram. Point P divides AB in the ratio 2 : 3 and point Q divides DC in the ratio 4:1. [2]

Prove that OC is half of OA.
Solution:
Given,

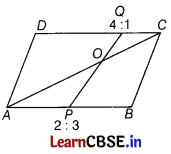
In ∆OAP and ∆OCQ,
∴ ∠AOP = ∠COQ [vertically opposite angles]
∴ ∠OAP = ∠OCQ [alternate angles]
⇒ ∆OAP ~ ∆OCQ [by AA similarity criterion]
∴ \(\frac{O A}{O C}=\frac{A P}{C Q}\) ………….(i) (1)
Given, \(\frac{A P}{P B}=\frac{2}{3}\) and \(\frac{D Q}{Q C}=\frac{4}{1}\)
\(\frac{A P}{A B}=\frac{2}{5}\) and \(\frac{Q C}{D C}=\frac{1}{5}\)
AP = \(\frac{2}{5}\) AB and QC = \(\frac{1}{5}\) DC (1/2)
From Eq. (i),
\(\frac{O A}{O C}=\frac{\frac{2}{5} A B}{\frac{1}{5} D C}\) = 2
[∵ ABCD is a parallelogram ∴ AB = CD]
⇒ OC = \(\frac{1}{2}\) OA (1/2)

Question 23.

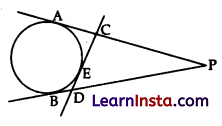
From an external point P, two tangents, PA and PB are drawn to a circle with centre O. At point E on the circle, a tangent is drawn to intersect PA and PB at C and D, respectively. If PA = 10 cm, find the perimeter of ΔPCD.
Solution:
PA ⇒ PB; CA = CE; DE = DB
[Tangents to a circle]
Perimeter of ΔPCD = PC + CD + PD
= PC + CE + ED + PD
= PC + CA + BD + PD
= PA + PB
Perimeter of APCD = PA + PA = 2PA
= 2(10) = 20 cm

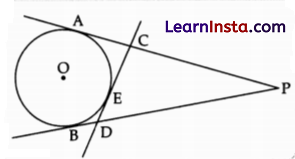
Detailed Answer:
P is a external point and PA and PB are drawn
then PA = PB = 10 cm

C, D are also external point and drawn CA, CE and ED, BD
Then ED = BD
AC = CE
Now, Perimeter of ΔPCD = PC + CD + PD
= PC + CE + ED + PD
= (PC + AC) + (BD + PD)
= PA + PB
= PA + PA
= 2PA
= 2 × 10cm
= 20cm

Question 24.
If tan(A + B) = √3 and tan(A – B) = \(\frac{1}{\sqrt{3}}\) ; 0°< A + B < 90°; A > B, find A and B. [2]
Or
Find the value of x
2 cosec²30° + x sin²60° – tan²30° = 10
Solution:
Given, tan(A + B) = √3 = tan 60°
and tan(A – B) = –\(\frac{1}{\sqrt{3}}\) = tan 30°
⇒ A + B = 60° and A – B = 30° (1)
⇒ 2A = 90°
⇒ A = 45°
and B = 15° (1)

Or

We have,
2 cosec²30° + x sin²60° – \(\frac{3}{4}\)tan²30° = 10
⇒ \(\frac{3}{4}\) =
⇒ 8 + \(\frac{3x}{4}\) – \(\frac{1}{4}\) = 10
⇒ \(\frac{3x}{4}\) = 2 + \(\frac{1}{4}\) = \(\frac{9}{4}\)
⇒ x = 3 (2)

Question 25.

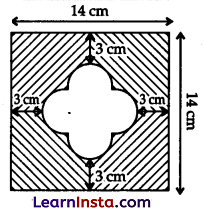
(A) With vertices A, B and C of ΔABC as centres, arcs are drawn with radii 14cm and the three portions of the triangle so obtained are removed. Find the total area removed from the triangle.
Solution:
Total area removed

Detailed Answer:

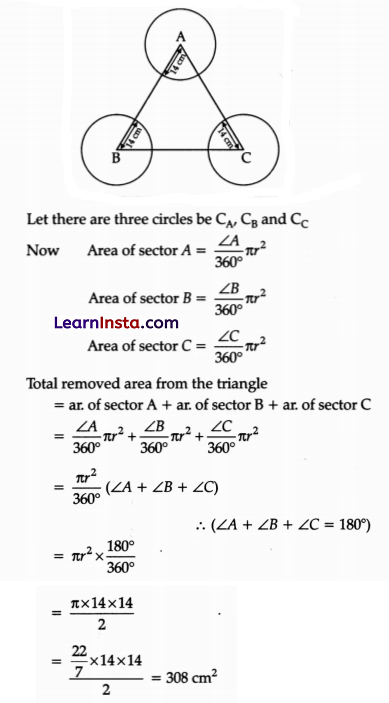
OR

(B) Find the area of the unshaded region shown in the given figure.
Solution:
The side of a square = Diameter of the semi-circle = a
Area of the unshaded region = Area of a square of side ‘a’ + 4(Area of a semi-circle of diameter ‘a’)
The horizontal/vertical extent of the white region
= 14 – 3 – 3 = 8cm
Radius of the semi-circle + Side of a square + Radius of the semi-circle = 8 cm
= 2(radius of the semi-circle) + side of a square
= 8cm
2a = 8cm
⇒ a = 4cm
Area of the unshaded region = Area of a square of side 4 cm + 4 (Area of a semi-circle of diameter 4 cm)
= (4)² + 4 × \(\frac{1}{2}\)π(2)²
= 16 + 8π cm²

Section – C (18 Marks)
Section C Consists of 6 questions of 3 marks each

Question 26.
The national Art convention got registrations from students from all parts of the country, of which 60 are interested in music, 84 are interested in dance and 108 students are interested in handicrafts. For optimum cultural exchange, organizers wish to keep them in a minimum number of groups such that each group consists of students interested in the same art form and the number of students in each group is the same. Find the number of students in each group. Find the number of groups in each art form. How many rooms are required if each group will be allotted a room? [3]
Solution:
Students interested in music = 60
Students interested in dance = 84
Students interested in handcrafts = 108
Prime factorization of 60, 84, and 108 are
60 = 2 × 2 × 3 × 5 = 2² × 3 × 5
84 = 2 × 2 × 3 × 7 = 2² × 3 × 7 (1)
and 108 = 2 × 2 × 3 × 3 × 3 = 2² × 3³ respectively.
HCF (60, 84, 108) = 2² × 3 = 12
Number of students in each group = HCF (60, 84, 108) =12
Number of groups in music = \(\frac{60}{12}\) = 5
Number of groups in dance = \(\frac{84}{12}\) = 7
Number of groups in handcrafts = \(\frac{108}{12}\) = 9
Rooms required if each group will be allotted a room
= Total number of groups = 5+7 + 9 = 21 (1)

Question 27.
If α, β are zeroes of quadratic polynomial 5x² + 5x + 1, find the value of
(1) α² + β²
(2) α^-1 + β^-1
Solution:
P(x) = 5x² + 5x + 1

Question 28.
The sum of a two-digit number and the number obtained by reversing the digits is 66. If the digits of the number differ by 2, find the number. How many such numbers are there? [3]
Or
Solve the following pair of linear equations by using the substitution method
2x + 3y = 11
2x-4y = -24
Solution:
Let the digit at unit place be x
and the digit at tens place be y.
∴ The two-digit number is 10y + x.
Now, the number obtained by reversing the digits = 10x + y (1)
According to the question,
(10y + x) + (10x + y) = 66
⇒ 11(x + y) = 66
⇒ x + y = 6 ……. (i)
Also, the digits of the number differ by 2
⇒ x – y = 2 ……….. (ii)
or y – x = 2 ……… (iii) (1)
On solving Eqs. (i) and (ii),
we get x = 4, y = 2
On solving Eqs. (i) and (iii),
we get y = 4, x = 2
∴ Two such numbers are there
They are 10(4) + 2 = 42
and 10(2) + 4 = 24 (1)

Or

Given, 2x + 3y = 11 ………..(i)
2x – 4y = – 24 ………..(ii)
From Eq. (i),
2x = 11 – 3y
Substituting the value of 2x in Eq. (ii), we get
11 – 3y – 4y = – 24
⇒ 11 – 7y = -24
⇒ 7y = 35
⇒ y = 5
Now, from 2x = 11 – 3y
Substituting y = 5 in the above relation, we get
2x = 11 – 3 × 5
= 11 – 15
= – 4
⇒ x = -2
Hence, x = – 2 and y = 5

Question 29.

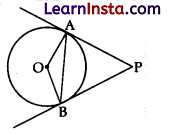
(A) PA and PB are tangents drawn to a circle of centre O from an external point P Chord AB makes an angle of 30° with the radius at the point of contact.
If length of the chord is 6 cm, find the length of the tangent PA and the length of the P radius OA.
Solution:
∠OAB = 30°
∠OAP = 90° [Angle between the tangent and the radius at the point of contact]
∠PAB = 90° – 30° = 60°
AP = BP
[Tangents to a circle from an external point]
∠PAB = ∠PBA
[Angles opposite to equal sides of a triangle]
In ΔABP ∠PAB + ∠PBA + ∠APB = 180°
[Angle Sum Property]
60° + 60° + ∠APB = 180°
∠APB = 60°
:. ΔABPis an equilateral triangle, where AP = BP = AB.
PA =6cm
In Right ΔOAP, ∠OPA = 30°
tan 30° = \(\frac{O A}{P A}\)
\(\frac{1}{\sqrt{3}}\) = \(\frac{O A}{6}\)
OA = \(\frac{6}{\sqrt{3}}\)
= 2√3 cm

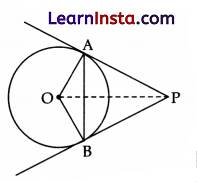
OR

(B) Two tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ∠PTQ = 2∠OPQ.
Solution:
Let ∠TPQ= 0
∠TPO = 90° [Angle between the tangent and
the radius at the point of contact]
∠OPQ = 90° – θ
TP = TQ
[Tangents to a circle from an external point]
∠TPQ = ∠TQP = θ
[Angles opposite to equal sides of a triangle]
In ΔPQT, ∠PQT + ∠QPT + ∠PTQ = 180°
[Angle Sum Property]
θ + θ + ∠PTQ = 180°
∠PTQ = 180° – 20
∠PTQ = 2(90°- θ)
∠PTQ = 2∠OPQ [using (1)]

Question 30.
If 1 + sin²θ = 3 sin θ cos θ, then prove that tan θ = 1 or \(\frac{1}{2}\). [3]
Solution:
Given, 1 + sin²θ = 3 sin θ cos θ
⇒ sin²θ + cos²θ + sin²θ = 3 sin θ cos θ
[∵ sin² A + cos² A = 1]
⇒ 2 sin²θ + cos²θ – 3 sin θ cos θ = 0
⇒ 2 sin²θ – 3 sin θ cos θ + cos²θ = 0
⇒ 2 sin²θ – 2 sin θ cos θ – sin θ cos θ + cos²θ = 0
⇒ 2 sin θ(sin θ – cos θ) – cos θ(sin θ – cos θ) = 0
⇒ (sin θ – cos θ) (2 sin θ – cos θ) = 0
⇒ sin θ – cos θ = 0 or 2 sin θ – cos θ = 0
⇒ sin θ = cos θ or 2 sin θ = cos θ
⇒ tan θ = 1 or tan θ = – 2

Question 31.
The length of 40 leaves of a plant are measured correct to nearest millimetre, and the data obtained is represented in the following table.

Length [in mm]	Number of leaves
118-126	3
127-135	5
136-144	9
145-153	12
154-162	5
163-171	4
172-180	2

Find the mean length of the leaves.
Solution:

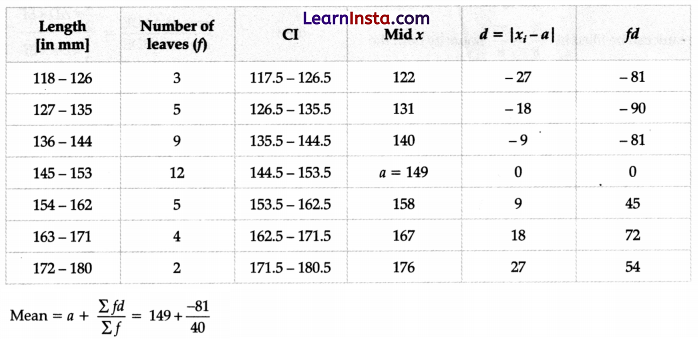
= 149 – 2.025 = 146.975
The average length of the leaves = 146.975

Section – D (20 Marks)
Section D consists of 4 questions of 5 marks each.

Question 32.
A motor boat whose speed is 18 km/h in still water takes 1 h more to go 24 km upstream than to return downstream to the same spot. Find the speed of stream. [5]
Or
Two water taps together can fill a tank in \(9 \frac{3}{8}\) h. The tap of larger diameter takes 10 h less than the smaller one to fill the tank separately.
Find the time in which each tap can separately fill the tank.
Solution:
Let the speed of the stream be x km/h.
Then, speed of the boat downstream = (18 + x) km/h
and speed of the boat upstream = (18 – x) km/h
Then, according to the question,
\(\frac{24}{18-x}\) – \(\frac{24}{18+x}\) = 1
⇒ 24(18 + x) – 24(18 – x) = (18 – x) (18 + x)
⇒ 24x + 24x = 324 – x²
⇒ x² + 48x – 324 = 0
⇒ x² + 54x – 6x – 324 = 0 (1)
⇒ x(x + 54) – 6(x + 54) = 0
⇒ (x + 54) (x – 6) = 0
∴ x = 6
[∵ x cannot be negative]
∴ Speed of stream = x = 6 km/h

Or

Let time taken by tap of smaller diameter be t h.
∴ Time taken by tap of larger diameter = (t -10) h
Given, time taken by both the taps to fill the tank
= \(9 \frac{3}{8}\) = \(\frac{75}{8}\)h
Portion of the tank filled by tap of smaller diameter in
1 h = \(\frac{1}{t}\)
Portion of the tank filled by tap of larger diameter in
1 h = \(\frac{1}{t-10}\)
Portion of the tank filled by both the taps in 1 h
= \(\frac{1}{\frac{75}{8}}\) = \(\frac{8}{75}\)
∴ \(\frac{1}{t}\) – \(\frac{1}{t-10}\) = \(\frac{8}{75}\)
⇒ \(\frac{(t-10)+t}{t(t-10)}\) = \(\frac{8}{75}\)
⇒ \(\frac{2t-10}{t^2-10t}\) = \(\frac{8}{75}\)
⇒ 150t – 750 = 8t² – 80t
⇒ 8t² – 230t + 750 = 0
⇒ 4t² – 115t + 375 = 0
⇒ 4t² – 100t – 15f + 375 = 0
⇒ 4t(t – 25) – 15(t – 25) = 0
⇒ (t – 25)(4t – 15) = 0
t = 25 or t = \(\frac{15}{4}\)
If t = 25, then time taken by tap of larger diameter
= 25 – 10 = 15 h
If t = \(\frac{15}{4}\), then time taken by tap of larger diameter
= \(\frac{15}{4}\) – 10 = \(– \frac{25}{4}\) which is not possible.
∴ Time taken by taps of smaller and larger diameter are 25 h and 15 h, respectively.

Question 33.
Image
(a) State and prove Basic Proportionality theorem.
Solution:
Statement: If a line is drawn parallel to one side of a triangle intersecting the other two side of distinct point, then the other two sides are divided in the same ratio.
Let ΔABC in which a line DE parallel to BC intersects AB at D and AC at E

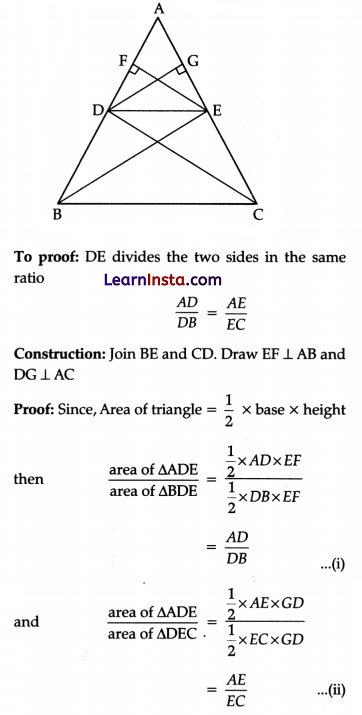
Since ΔBDE and ΔDEC lie between the same parallel DE and BE and are on the same base DE.
We have area of ΔBDE = area of ΔDEC …(iii)
From eqs. (i), (ii) and (iii) we get
\(\frac{A D}{D B}\) = \(\frac{A E}{E C}\) Hence Proved

(b) In the given figure ∠CEF = ∠CFE. F is the midpoint of DC.
Prove that \(\frac{A B}{B D}\) = \(\frac{A E}{F D}\).

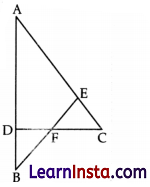
Solution:

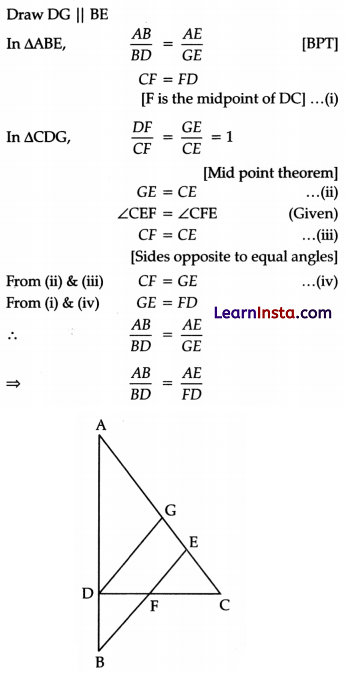
Question 34.
Water is flowing at the rate of 15 km/h through a pipe of diameter 14 cm into a cuboidal pond which is 50 m long and 44 m wide. In what time will the level of water in pond rise by 21 cm?
What should be the speed of water, if the rise in water level is to be attained in 1 h?
Or
A tent is in the shape of a cylinder surmounted by a conical top. If the height and radius of the cylindrical part are 3 m and 14 m, respectively and the total height of the tent is 13.5 m, find the area of the canvas required for making the tent, keeping a provision of 26 m² of canvas for stitching and wastage. Also, find the cost of the canvas to be purchased at the rate of ₹ 500 per m². [5]
Solution:
Given, the diameter of cylindrical pipe = 14 cm
⇒ Radius of cylindrical pipe (r) = 7 cm
Also, length of the pond (l) = 50 m,
Breadth of the pond (b) = 44 m,

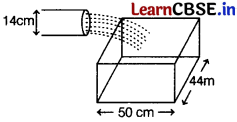
Height of the water level in the pond (h) = 21 cm
Lett h be the time taken to raise the level of water by 21 cm.
Length of the water column in f h = (15 × t) km
= 15000t m³
Volume of water column that come out from cylindrical pipe in t h
= π × \(\left(\frac{7}{100}\right)^2\) × 15000t
∴ Volume of water column =Volume of cuboid with height h
⇒ π × \(\left(\frac{7}{100}\right)^2\) × 15000t = 50 × 44 × \(\frac{21}{100}\)
⇒ \(\frac{22}{7}\) × \(\frac{7^2}{10}\) × 15t = 22 × 21
⇒ 7 × 15t = 210 ⇒ t = 2 h
Hence, in 2 h, level of water in pond rise by 21 cm.
Let v km/h be the speed of water.
Length of the water column in 1 h = v km = (v × 1000)m
∴ Volume of water column
= π × \(\left(\frac{7}{100}\right)^2\) × v × 1000
= \(\frac{22 \times 7 \times v}{10}\) m³
∴ \(\frac{22 \times 7 \times v}{10}\) = 50 × 44 × \(\frac{21}{100}\)
⇒ v = 30 km/h
Hence, speed of water is 30 km/h.

Or

Given, total height of the tent = 13.5 m
and radius of the base of the tent=14 m
As shown in figure,
Height of the cone, = Total height – Height of cylinder.
= 13.5 – 3 = 10.5m

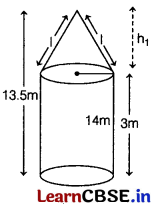
Radius of cone and radius of cylinder r = 14 m
Slant height / of cone = \(\sqrt{h_1^2 + r^2}\)
=\(\sqrt{(105)^2 + (14)^2}\) = 17.5 m
Curved surface area of cone = πrl
= π × 14 × 17.5 = 245 π m²
Curved surface area of cylinder = 2πrh²,
where h² is height of cylinder
= 2π × 14 × 3 = 847t m²
∴ Area of canvas required = 245π + 84π + 26
= 245 × \(\frac{22}{7}\) + 84 × \(\frac{22}{7}\) + 26
= 770 + 264 + 26= 1060 m²
Cost of canvas = ₹ (500 x 1060) = ₹ 530000

Question 35.
The median of the following data is 50. Find the values of ‘p’ and ‘q’, if the sum of all frequencies is 90. Also find the mode of the data.

Marks obtained	Number of students
20-30	p
30-40	15
40-50	25
50-60	20
60-70	q
70-80	8
80-90	10

Solution:

Marks obtained	Number of students	Cumulative frequency
20-30	p	p
30-40	15	p + 15
40-50	25	p + 40
50-60	20	p + 60
60-70	q	p + q + 60
70-80	8	p + q + 68
80-90	10	p + q + 78
	90

p + q + 78 = 90
p + q = 12

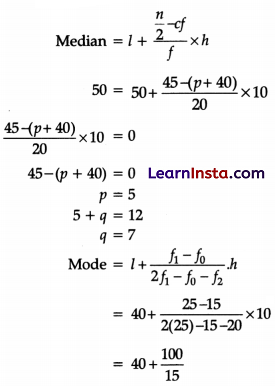
= 40 + 6.67
= 46.67

Section – E (12 Marks)
Case study based questions are compulsory

Question 36.
Manpreet Kaur is the national record holder for women in the shot-put discipline. Her throw of 18.86 m at the Asian Grand Prix in 2017 is the biggest distance for an Indian female athlete.
Keeping her as a role model, Sanjitha is determined to earn gold in the Olympics one day.
Initially, her throw reached 7.56 m. only. Being an athlete in school, she regularly practiced both in the mornings and in the evenings and was able to improve the distance by 9 cm every week.
During the special camp for 15 days, she started with 40 throws and every day kept increasing the number of throws by 12 to achieve this remarkable progress

(i) Flow many throws Sanjitha practiced on 11th day of the camp? [1]
(ii) What would be Sanjitha’s throw distance at the end of 6 months? [2]
Or
When will she be able to achieve a throw of 11.16m? [2]
(iii) How many throws did she do during the entire camp of 15 days ? [1]
Solution:
(i) Number of throws on first day a = 40
Number of throws on second day = 40 + 12 = 52
AP formed by number of throws is 40, 52, (52 + 12)…
(I day) (II day) (III day)
Here, common difference,
d = 12
Number of throws she practiced on 11th day
a₁₁= a + (11 – 1)d
= 40 + 10 × 12 = 160
Distance of Sanjitha’s throw on first day,
a = 7.56 m
Distance of Sanjitha throw after a week
= (7.56 + 0.09) m = 7.65 m
AP formed by the distances is
7.56, 7.65, 7.65 + (0.09) ………
(I week) (II week) (III week)
Here, common difference, d = 0.09 m
Sanjitha’s throw distance at the end of 6 months (i.e. 26 weeks)
a₂₆ = a + (26 – 1)d
= 7.56 + 25 × 0.09
= 7.56 + 2.25
= 9.81 m

Or

Here, a_n = 11.16 = a + (n – 1) d
⇒ 7.56 + (n – 1)0.09 = 11.16
⇒ n – 1 = \(\frac{3.6}{0.09}\) = n = 41
∴ On 41st week, she will be able to achieve a throw of 11.16 m.

(iii) Total throws done by her during the entire camp of
15 days = S_n = \(\frac{n}{2}\) {2a + (n – 1) d}
where n is total number of terms
⇒ S₁₅ = \(\frac{15}{2}\) [2 × 40 + 14 × 12]
= 15[40 + 84] = 15 × 124 = 1860

Question 37.
Tharunya was thrilled to know that the football tournament is fixed with a monthly time frame from 20th July to 20th August 2023 and for the first time in the FIFA Women’s World Cup’s history, two nations host in 10 venues. Her father felt that the game can be better understood if the position of players is represented as points on a coordinate plane.

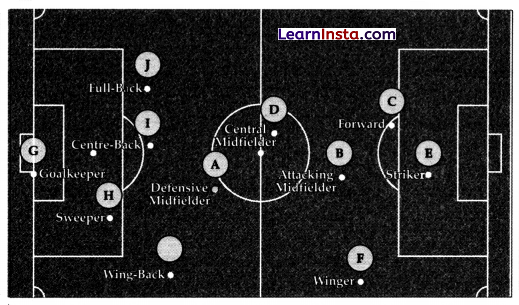
(i) At an instance, the mid-fielders and forward formed a parallelogram. Find the position of the central mid-fielder (D) if the position of other players who formed the parallelogram are: A(1, 2), B(4, 3) and C(6,6).
Solution:
Let D be (a, b), then
Mid point of AC = Midpoint of BD

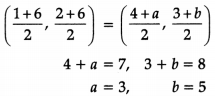
Central mid-fielder is at (3, 5).

(ii) Check if the Goal keeper G(-3, 5), Sweeper H(3, 1) and Wing-back K(0, 3) fall on a same straight line.
OR
Check if the Full-back J(5, -3) and centre-back I(-4, 6) are equidistant from forward C(0, 1) and if C is the mid-point of J.
Solution:

C is NOT the mid-point of IJ.

(iii) If Defensive mid-fielder A(1, 4), Attacking mid-fielder B(2, -3) and Striker E(a, b) lie on the same straight line and B is equidistant from A and E, find the position of E.
Solution:
A, B and E lie on the same straight line and B is equidistant from A and E.
⇒ B is the mid-point of AE
\(\left(\frac{1+a}{2}, \frac{4+b}{2}\right)\) = (2, -3)
1 + a = 4; a = 3;
4 + b = -6; b = -10;
E is (3, -10).

Question 38.
One evening, Kaushik was in a park. Children were playing cricket. Birds were singing on a nearby tree of height 80m. He observed a bird on the tree at an angle of elevation of 45°.
When a sixer was hit, a ball flew through the tree frightening the bird to fly away. In 2 s, he observed the bird flying at the same height at an angle of elevation of 30° and the ball flying towards him at the same height at an angle of elevation of 60°.

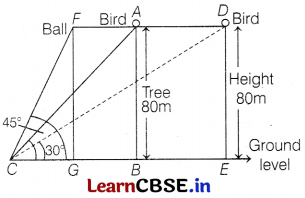
(i) At what distance from the foot of the tree was he observing the bird sitting on the tree? [1]
(ii) How far did the bird fly in the mentioned time? [2]
Or
After hitting the tree, how far did the ball travel in the sky when Kaushik saw the ball? [2]
(iii) What is the speed of the bird in m/min, if it had flown 20(√3 + 1) m? [1]
Solution:
(i) In right-angled ∆ABC,
tan 45° = \(\frac{A B}{B C}\)
⇒ BC = AB = 80m …(i)
[∵ AB = 80 m and tan 45° = 1]
∴ Required distance = 80 m

(ii) In right-angled ∆DEC,

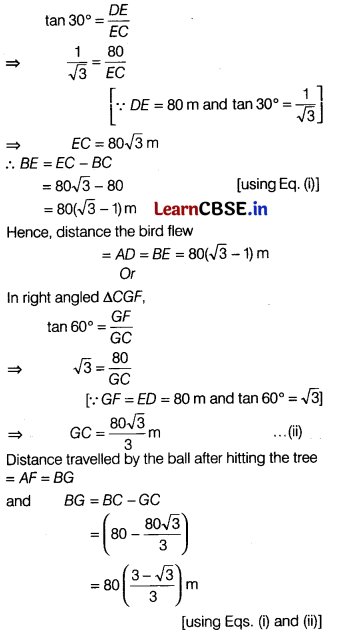
(iii) Speed of the bird = \(\frac{\text { Distance }}{\text { Time }}\)
\(\frac{20(\sqrt{3}+1)}{2}\) m/s
= 10(√3 + 1) m/s
= 600(√3 + 1) m/min
[∵1s = \(\frac{1}{60}\) min 60]